Home >> Optics, concave mirrors

Basic ray diagram

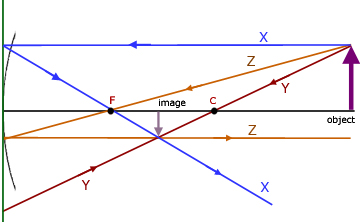
The basic ray diagram for a concave mirror introduces a number of important terms:

aperture - the diameter of the circular mirror

pole - where the principal axis meets the mirror surface

centre of curvature - the centre of the sphere that the mirror forms part

radius of curvature (r) - radius of the sphere

principal axis - the line through the centre of curvature and the pole of the mirror

focal length (f) - equal to half the radius of curvature f = r/2

back to top

Ray diagrams

Ray diagrams are constructed by taking the path of three distinct rays from a point on the object:

X) a ray parallel to the principal axis reflected through F (the principal focus)

Y) a ray passing through C which is then reflected back along its original path

Z) a ray passing through F, which is then reflected parallel to the principal axis

note - the concave mirror is considered to be so thin as to be represented by a vertical line

The image types and positions for a concave mirror are very similar to those of a convex lens.

object between F and the lens

The image is upright, virtual and magnified.

object at F

The image is formed at infinity from parallel rays that do not converge. Therefore no image is formed.

object between F and C

The image is real, inverted and magnified.

object at C

The image is formed at C.
The image is inverted, real and the same size as the object.

object at infinity

The image is formed at the focal point of the lens. It is real, inverted and diminished in size.

back to top

Proof of r = 2f

The angle of incidence equals the angle at C (corresponding angles).

From the diagram it is apparent that XF does not equal PF. However, when point X is closer to the principal axis, XF approaches the size of PF. So for rays close to the principal axis we can say that XF = PF.

In other words,

XC = PF + FC

r = f + f

r = 2f

back to top

The Mirror Formula

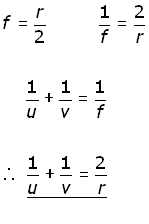
The sign convention, 'real is positive' is used:

1) focal length (f) and radius of curvature (r) are both positive for concave mirrors

2) distances to real images and real objects are positive

3) distances to virtual images and virtual objects are negative

back to top

Caustic Curves

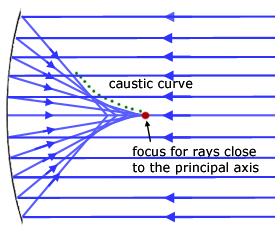
The caustic is the name given to the region of a concave mirror where parallel rays of light come to different foci. This happens for rays away from the principal axis. The further away they are, the closer is the focus to the mirror.

back to top

Parabolic mirrors

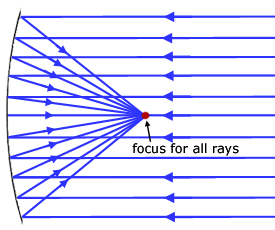
A parabolic mirror produces one focus for all rays parallel to the principal axis, irrespective of their distance from it. Parabolic mirrors have uses in telescopes, solar furnaces, and car headlights/torches/floodlights etc.

back to top

[ About ] [ FAQ ] [ Links ] [ Terms & Conditions ] [ Privacy ] [ Site Map ] [ Contact ]