Home >> Waves, diffraction of light

Basic concepts

Diffraction is the bending of light at an edge as a result of the superposition of wavelets from a plane wavefront.

back to top

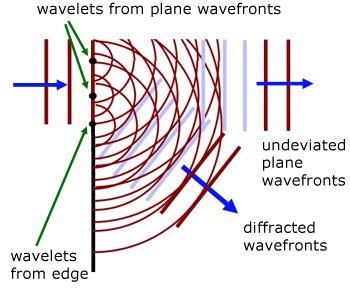
Single Slit

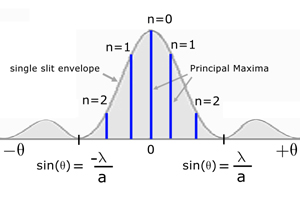
The diffraction pattern is graphed in terms of intensity and angle of deviation from the central position.

Note that the central maximum is twice the width of other maxima and that all these have the same width.
The diagram and image give a false impression regarding the relative brightness of fringes. The secondary maxima are considerably dimmer than the central maximum(4.7% of the brightness).

To frame an equation that predicts outcomes from this waveform, we must re-visit work on the superposition of waves.
The dark fringes(minima) are where pairs of light waves are in anti-phase and cancel out.

Consider pairs of light waves interfering with each other across the width of the slit.
The first light wave (1) in the upper half of the slit interferes destructively with the light wave (k) from the middle of the slit.
Then,

light wave 2 interferes with wave k+1,
light wave 3 interferes with wave k+2,

and so on, until light wave k+1 interferes with light wave 2k. For each pair of light waves the phase difference is half a wave length and the vertical distance between wave points is a/2.

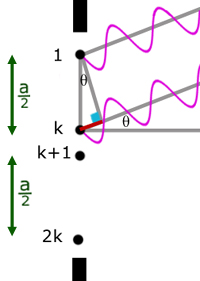
So, for the first minimum, we look at the first and last waves in this segment(a/2) with a phase difference of λ/2 (π - pi radians).
Using simple trigonometry, the path difference (in red) is equal to a/2 sin(θ).
Equating,

Unfortunately lack of space precludes the derivation of further minima.
As a general rule, the angular positions θ of the minima are given by:

In practice the slit width (a) is much larger than the wavelength (λ) of light used.          a >> λ

Rearranging our single slit equation, with n=1 for the first minimum,

As a result of the ratio λ/a being very small, sin(θ) ≈ θ in value.

So the equation becomes:

back to top

Multiple slit diffraction

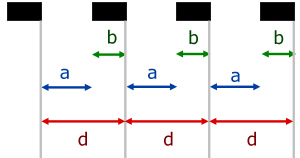
N - the no. of clear slits drawn on an opague glass slide
a - the width of a clear area
b - the width of an opague area
d - width of a clear and an opague area
L - length of glass slide

d = a + b

N=L/d

The diffraction patterns below are obtained by varying N.

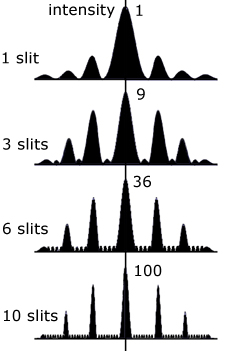
Note that with increasing N,

1. as the intensities of the principal maxima increase the intensities of the subsidiary maxima decrease
2. the sharpness of the principal maxima increases
3. the angular position of maxima remains the same
4. the absolute intensity of maxima increases

For a particular value of N, the effect of altering the sum a+b is to:

1. alter the angular positions of the principal maxima
2. alter the relative intensities of principal maxima

back to top

Diffraction grating

The clear spaces on a diffraction grating act as equally spaced slits.

So light rays diffracted at the same angle (θ) and in phase with each other will interfere constructively. Whenever this happens a bright fringe called a principal maxima is produced.

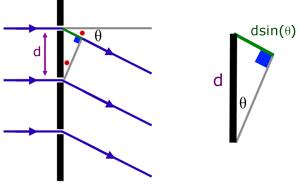
The path difference between successive light rays must therefore be a whole number (n) of wavelengths (λ).

Using simple trigonometry, if d is the distance between slits, then the path difference is dsin(θ).

Hence,

So discrete bright fringes(principal maxima) are produced at specific angles for particular wavelengths of light.

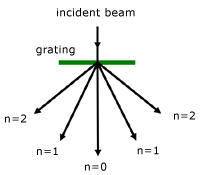
It must be noted that the distribution of light intensity across a diffraction display remains that of a single slit.

Grating and Prism spectra compared

From intermediate physics you may remember 'red rays refract least', illustrated by the prism diagram below.

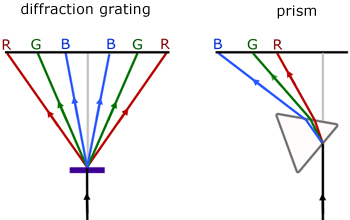
However, for diffraction gratings the deviation of coloured rays is the reverse.
If we rearrange our diffraction grating equation to make wavelength the subject, making n=1 for the first principal maxima to the right or left.

We can see that when wavelength λ is large(eg red light) sin(θ) is large, because λ and sin(θ) are directly proportional to each other. So θ is also large.
Now consider the case when wavelength λ is small (eg blue light). By proportion, sin(θ) will also be small. So θ will be small.

back to top

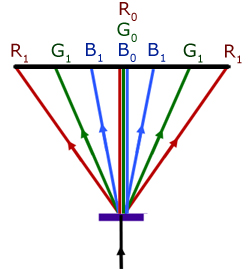
Grating spectra

When using a diffraction grating to examine spectra and measure wavelength only the principal maximum with n=1 is used.
The first principal maximum at n=0 is a bright fringe with all wavelengths mixed.
So if white light, made from a mixture of pure red, green and blue wavelengths were examined, the central fringe would be pure white.
Examining the principal maxima on either side, with order n=1 we find different coloured fringes deviated at different angles. Each fringe has a fair degree of brightness.
Note that the principal maxima for n=2 have greater deviations (right and left) and are considerably dimmer.

back to top

[ About ] [ FAQ ] [ Links ] [ Terms & Conditions ] [ Privacy ] [ Site Map ] [ Contact ]